
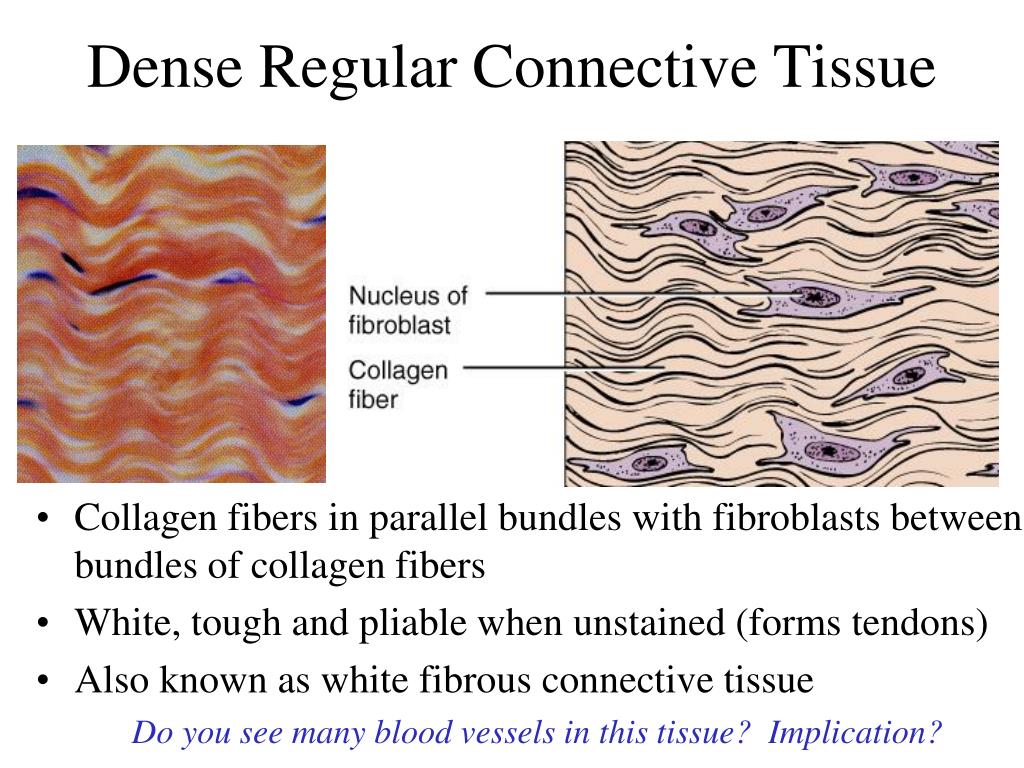
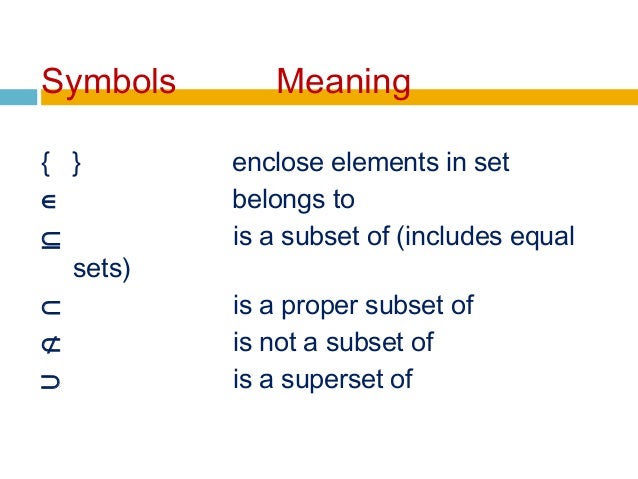
In topology and related areas of mathematics, a subset A of a topological space X is said to be dense in X if every point of X either belongs to A or else is arbitrarily "close" to a member of A - for instance, the rational numbers are a dense subset of the real numbers because every real number either is a rational number or has a rational number arbitrarily close to it (see Diophantine approximation). JSTOR ( February 2010) ( Learn how and when to remove this template message).Unsourced material may be challenged and removed. and only if for every open set V with x V, we have fk(x) V for some positive. Please help improve this article by adding citations to reliable sources. To prove that each orbit is dense, we will show first that no point. The following properties hold: (1) An open set is. But T is a constructible set, being the disjoint union of two locally closed sets A2 f x 0g(open) with f(0 0)g(closed). Our main results are: (1) period set of an SFT (or soc shift) is a non empty set of the form FN G, where F and G are. The set T (A2 f x 0g) f(0 0)g, being dense in A2 is not locally closed (Z would have to contain A2 f x 0g), hence would be equal to A2 and T is not open). An innite subshift is said to be Devaney chaotic (simply we say chaotic), if it is transitive and has a dense set of periodic points. X is separable if it has a countable dense set. if for any nonempty open sets U,V in X, there exists n N such that n(U) V. Let V be an open set containing x such that y V implies d(y, fn(y)) >. This article needs additional citations for verification. D X is dense if it intersects every non-empty open subset of X. cofinitely sensitive, (iv) any syndetically transitive, infinite subshift is.


 0 kommentar(er)
0 kommentar(er)
